Computer Graphics
There's a total of 23 articles.
Flat shading


Diffuse shading

As an example on the picture (credits to Marc Kleen) we see a real life car with a matte coating which we want to emulate using the Lambertian shading model.

Introduction to surface shading


Quaternions


Culling & Clipping
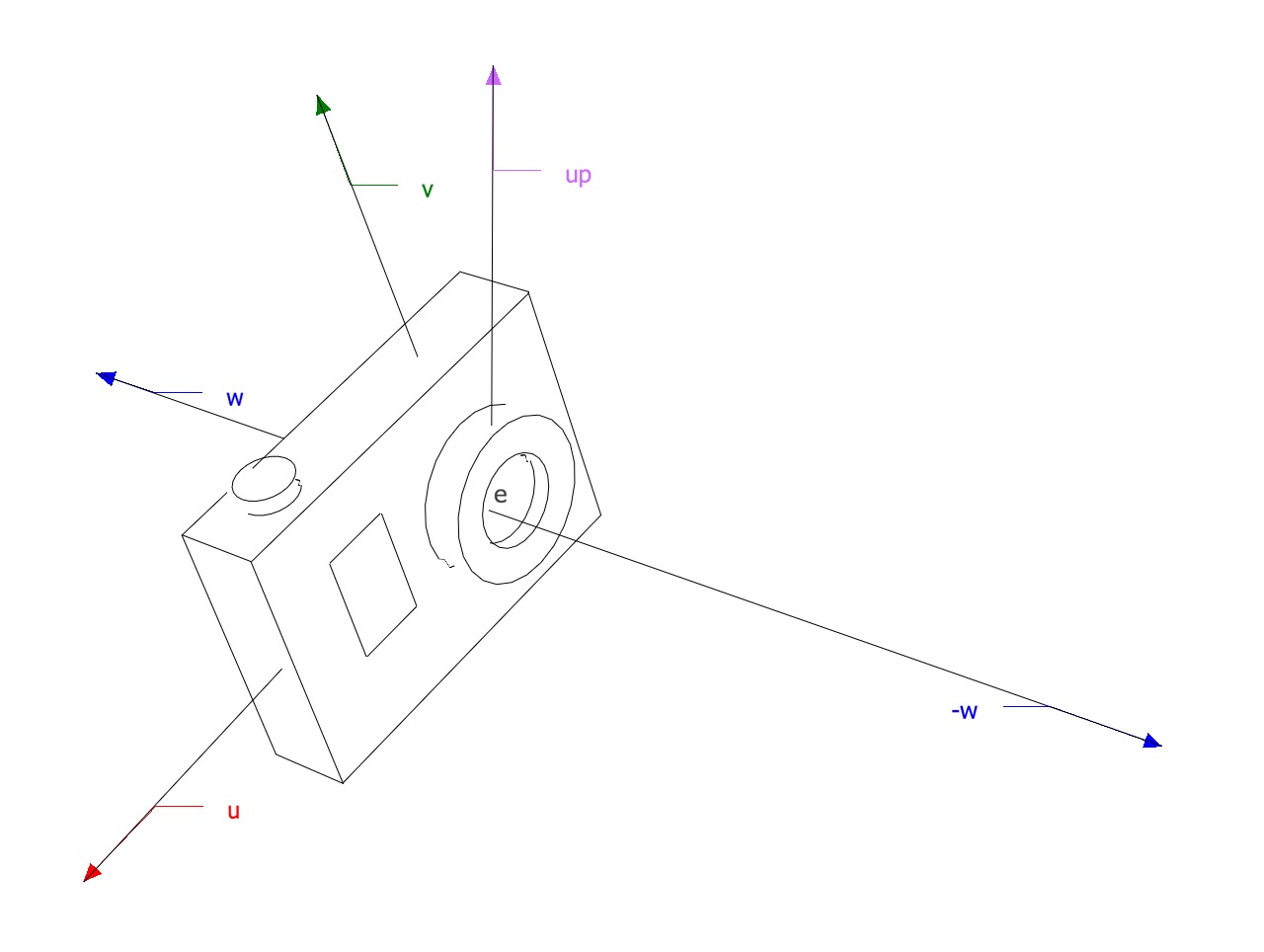
The math behind culling and clipping and how it’s related with the camera and with what it sees.
- Culling is a process where geometry that’s not visible from the camera is discarded to save processing time.
- Clipping is a process that removes parts of primitives that are outside the view volume (clipping against the six faces of the view volume).

Vector spaces
A vector space is a set whose elements are called “vectors” (denoted as $\v{v}$ or $\mathbf{v}$) which have two operations defined on them: addition of vectors and multiplication of an scalar by a vector.
This article covers some examples of vector spaces, basis of vectores spaces and linear maps.

Transformation matrix to transform objects from NDC coordinates to screen coordinates (viewport transform)

One matrix transformation in the 3D to a 2D transformation pipeline is the viewport transform where objects are transformed from normalized device coordinates (NDC) to screen coordinates (SC).
In short it's the transformation of numbers in the range [-1, 1] to numbers corresponding to pixels on the screen, which is a linear mapping computed with linear interpolation.
In this article I cover the math behind the generation of the viewport transformation matrix.

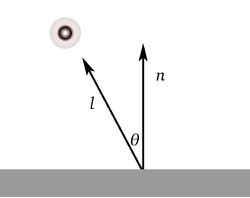
Normals

Eigenvalues and eigenvectors


Ray Tracing
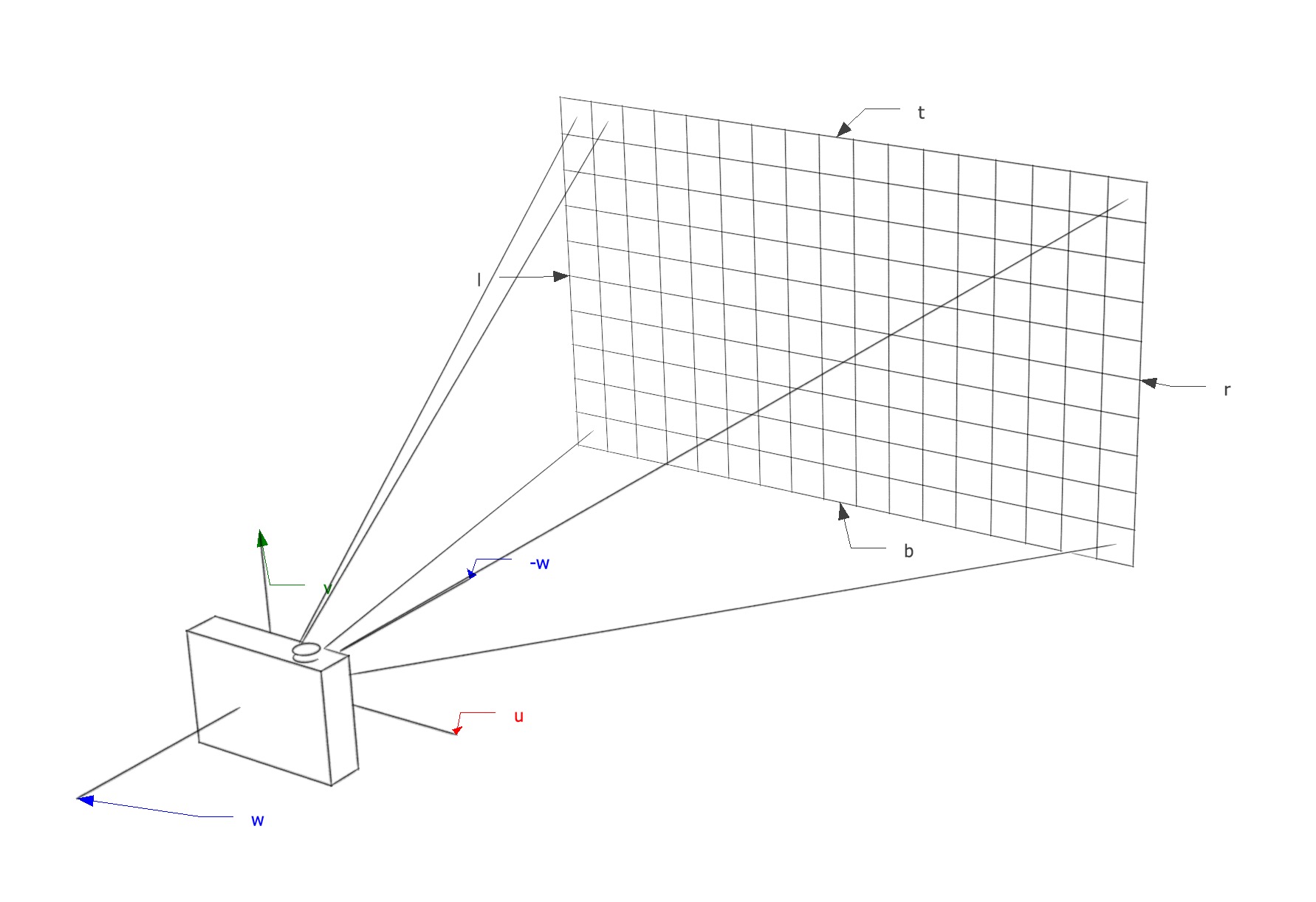

Rendering


Transformation matrix for projection of 3D objects into a 2D plane (projection transform)
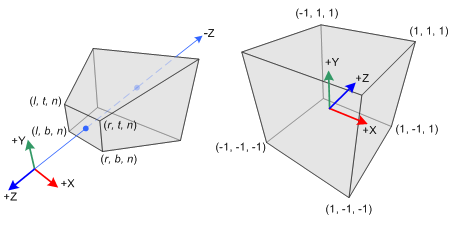
In Computer Graphics 3D objects created in an abstract 3D world will eventually need to be displayed in a screen, to view these objects in a 2D plane like a screen objects will need to be projected from the 3D space to the 2D plane with a transformation matrix.
In this article I cover two types of transformations: Orthographic projection and Perspective projection and analyze the math behind the transformation matrices.

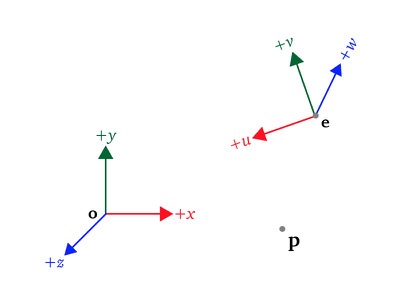
Transformation matrix to transform 3D objects from World Space to View Space (View transform)

One matrix transformation in the 3D to a 2D transformation pipeline is the view transform where objects are transformed from world space to view space. a transformation matrix.
In this article I cover the math behind the generation of this transformation matrix.

Combining Matrix Transformations
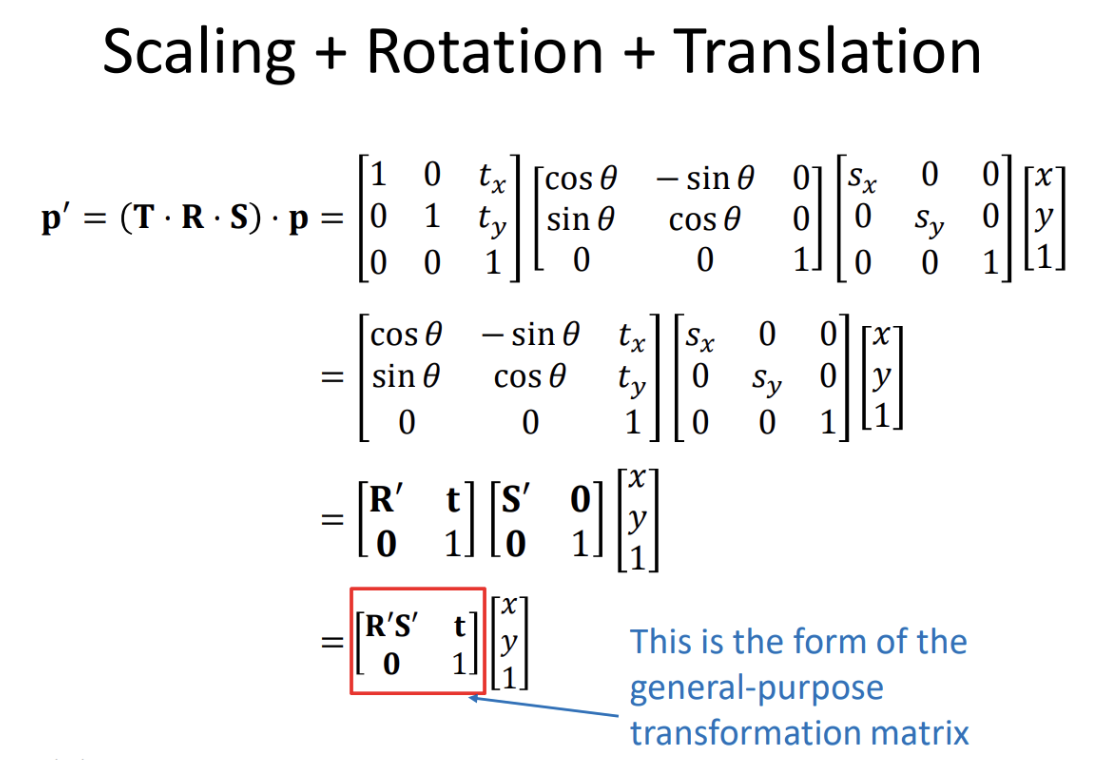

Perspective projection

This article covers the math behind it and how to generate the transformation matrix to achieve the transformation.

Orthographic projection

This article covers the math behind it and how to generate the transformation matrix to achieve the transformation.

Translating objects with a Transformation Matrix
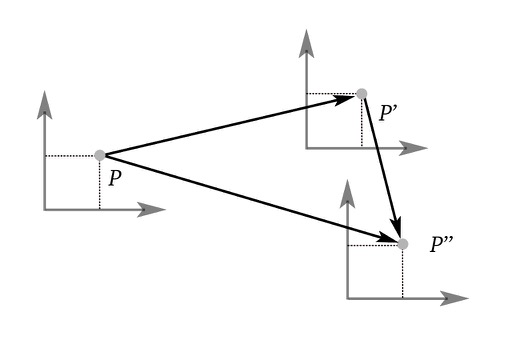

Euler angles

Euler angles are a way to describe the orientation of a rigid body with 3 values, these values represent 3 angles:
- yaw - Rotation around the vertical axis
- pitch - Rotation around the side-to-side axis
- roll - Rotation around the front-to-back axis

Shearing objects with a Transformation Matrix
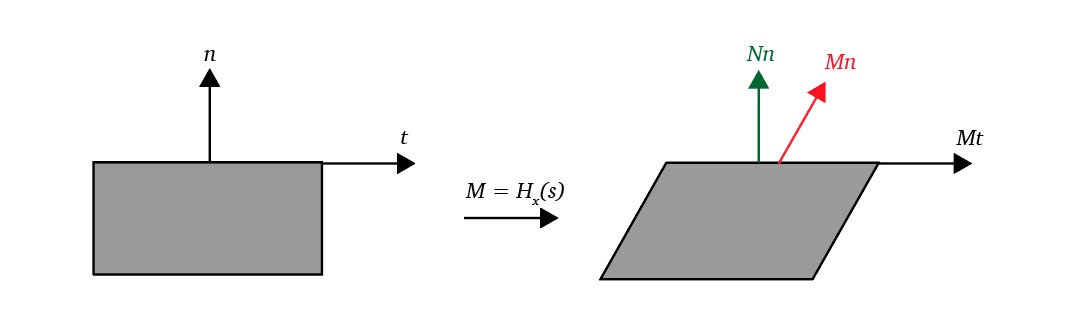

Introduction to rotation for computer graphics

The basics of rotation in 2d and 3d for computer graphics with a focus on 3d rotation about cardinal axes and 3d rotation with quaternions.
For quaternions, please also look at https://eater.net/quaternions amazing animations!

Scaling objects with a Transformation Matrix
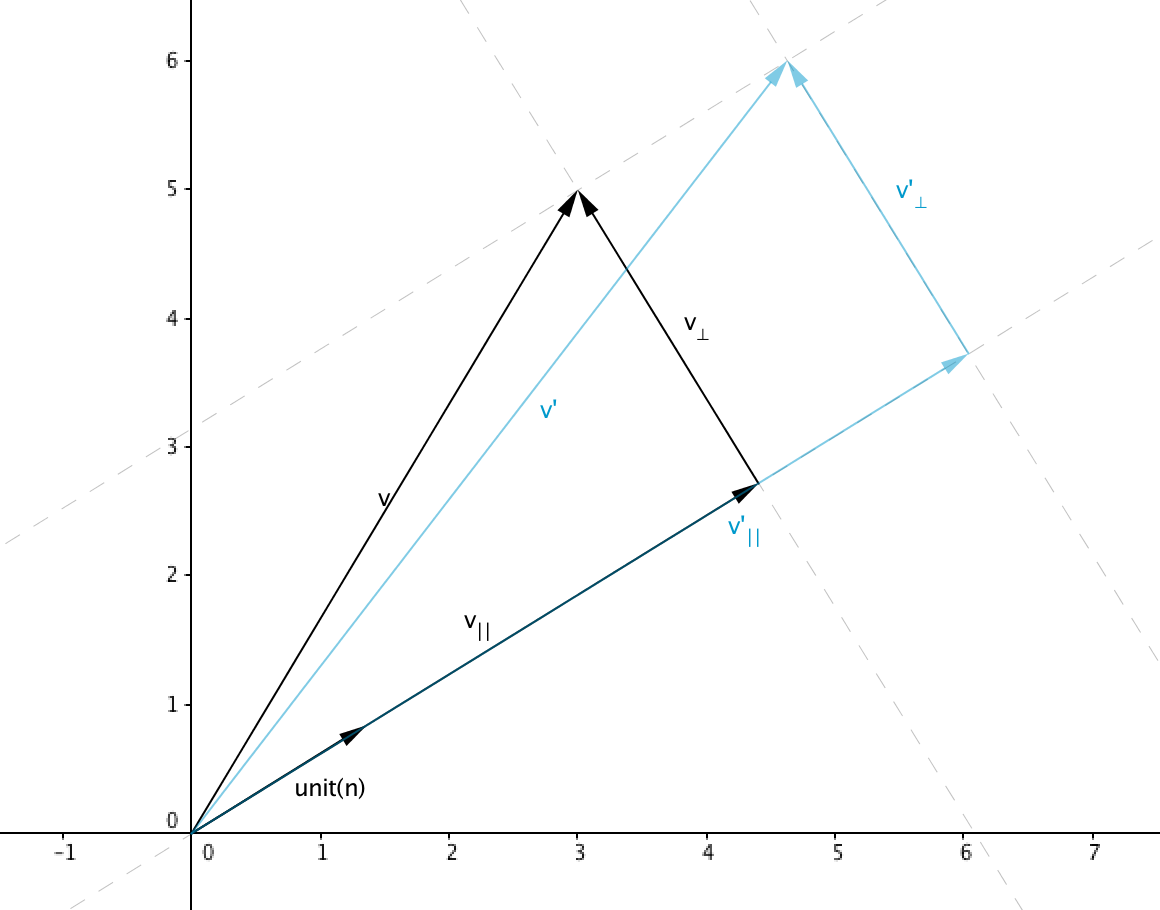

Transformation matrix


Coordinate systems and transformations between them