Math
There's a total of 25 notes.
Bayesian Networks


Divisibility

Let $a,b \in \mathbb{Z}$, we say that $a$ divides $b$, written $a \given b$, if there’s an integer $n$ so that: $b = na$. If $a$ divides $b$ then $b$ is divisible by $a$ and $a$ is a divisor or factor of $b$, also $b$ is called a multiple of $a$.
This article covers the greatest common divisor and how to find it using the euclidean algorithm, the extended euclidean algorithm to find solutions to the equation $ax + by = gcd(a, b)$ where $a, b$ are unknowns.

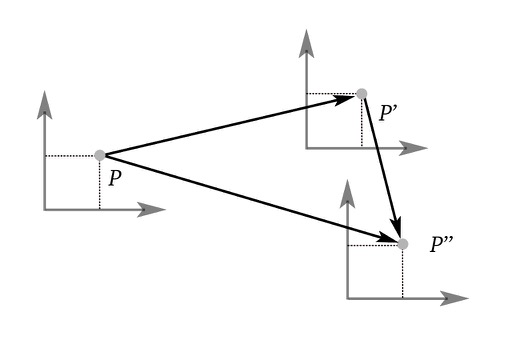
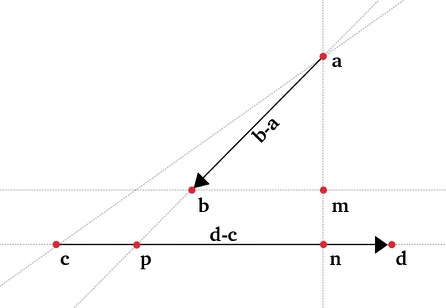
Affine spaces

Geometric tests

Eigenvalues and eigenvectors


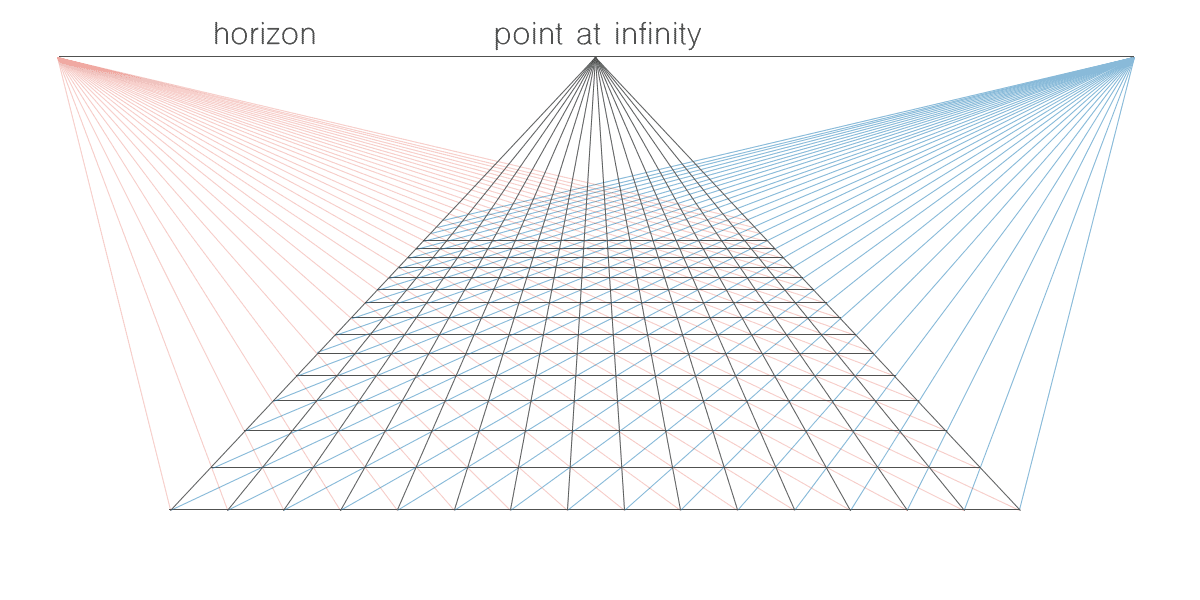
Projective space

Quaternions

Quaternions are a set of 4-dimensional vectors that are used to represent rotations in computer graphics, they were discovered by William Hamilton as an extension of 2d complex numbers to a 3d equivalent.
This article covers the definition of a quaternion, its notation and operations.

Complex numbers

Imaginary numbers were invented to solve problems for equestions with no real roots, complex numbers extend imaginary numbers by adding a real number.
This article covers the definition of complex numbers, operations such as addition, product, norm, conjugate, inverse and square root. Finally, this article covers the geometric and polar representations of complex numbers.

Integer Factorization

Integer factorization is the process of decomposing a composite number into a product of smaller integers, if these integers are restricted to be prime numbers then the process is called prime factorization.
This article covers factorization using trial division and fermat factorization through Pollard's Rho algorithm and using the sieve of eratosthenes.

Divisor Function


Primality Test

A prime number is a natural number greater than $1$ which has no positive divisors other than $1$ and itself.
This article covers different algorithms for checking if a number is prime or not including a naive test, the erathostenes sieve, the euler primality test and the miller-rabin primality test.

Prime factors of a factorial


Special factorial modulo p


Discrete Logarithm


Chinese Remainder Theorem

The chinese remainder theorem (CRT) is a theorem that deals with finding a solution to a system of congruences.
This article covers the defition of the CRT and an example implementation in C++.

Modular Arithmetic

Modular arithmetic is a type of arithmetic that deals with integers and remains within a fixed range of values. It involves performing arithmetic operations such as addition, subtraction, multiplication, and division, but with the added concept of a “modulus” or a “mod” value.
This article covers the definition a congruence relation, and some of its properties like addition, multiplication, exponentiation and inverse. Next I show how we can use the extended euclidean algorithm to find the modular multiplicative inverse in a general case and in the case of coprime numbers.

Extended Euclidean Algorithm


Binary Exponentiation


Erathostenes Sieve


Euclidean Algorithm


Euler's phi function


Derivative
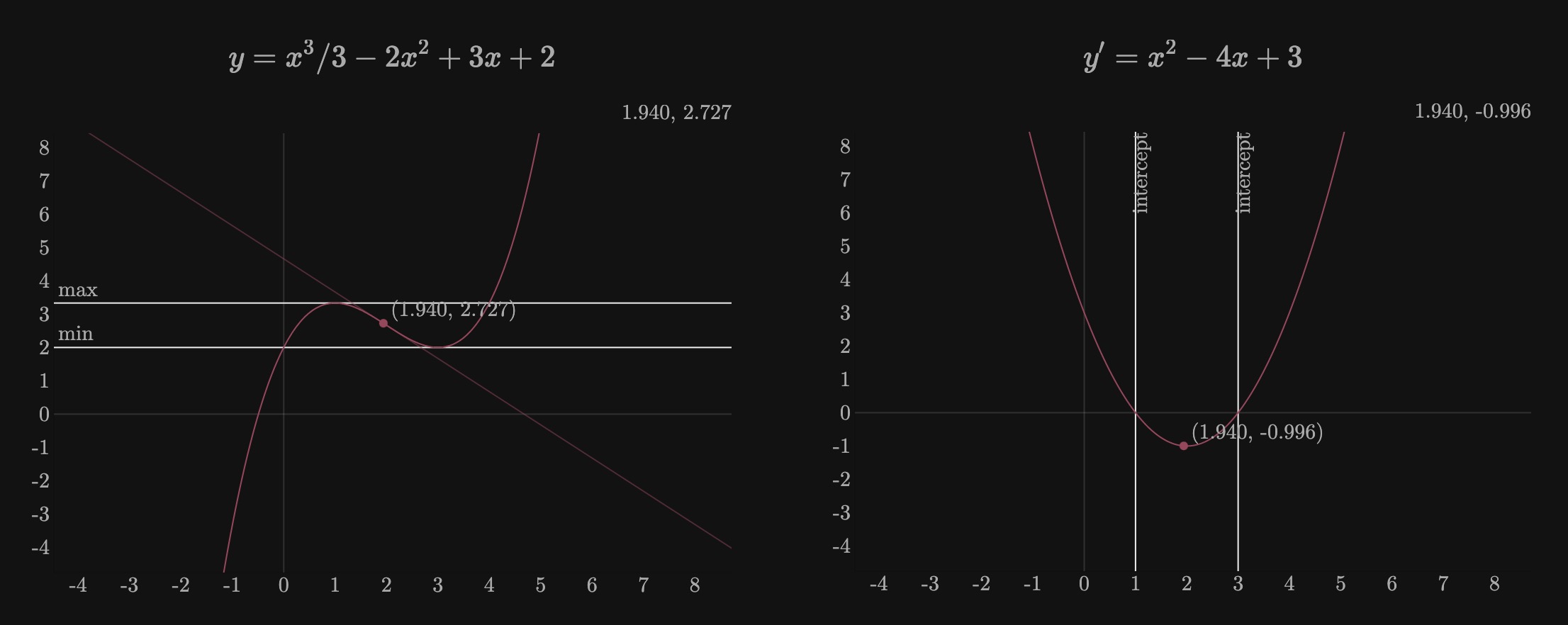
The derivative is a concept that represents the rate of change or the slope of a function at a particular point. It is a fundamental concept in calculus and is used to analyze how a function changes with respect to its input as the input changes very slightly.
This article covers physical and geometric interpretation of the derivative as well as some applications like finding maxima and minima in a function and newton-raphson.

Integral
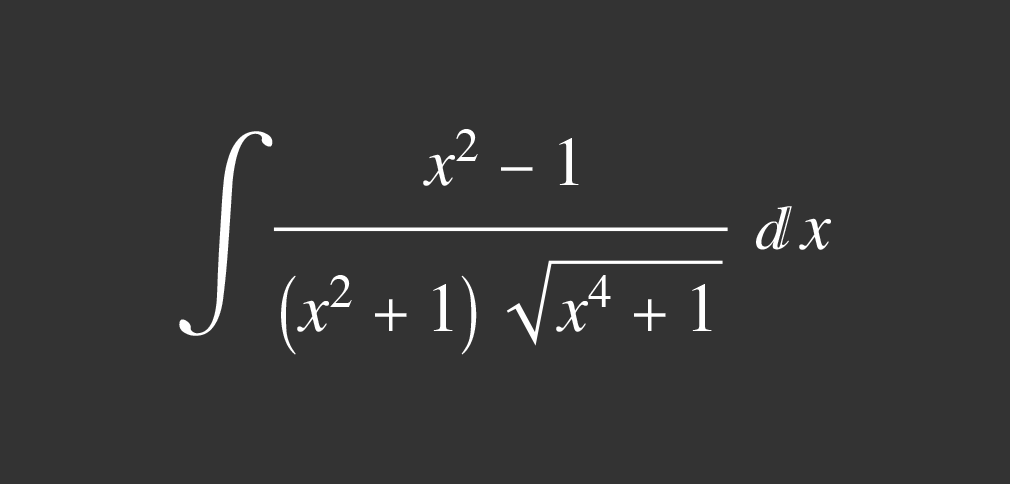

Taylor's Theorem and Infinite Series


Introduction to Calculus